 Vecto trong không gian - Từ điển môn Toán 12
Vecto trong không gian - Từ điển môn Toán 12 Cách phân tích một vecto theo các vecto cho trước - Toán 12
1. Cách phân tích một vecto theo các vecto cho trước
Để phân tích vecto \(\overrightarrow x \) theo các vecto \(\overrightarrow {{x_1}} \), \(\overrightarrow {{x_2}} \),…, \(\overrightarrow {{x_n}} \) cho trước, ta chèn các điểm để xuất hiện \(\overrightarrow {{x_1}} \), \(\overrightarrow {{x_2}} \),…, \(\overrightarrow {{x_n}} \) và biến đổi đến khi nào chỉ còn \(\overrightarrow {{x_1}} \), \(\overrightarrow {{x_2}} \),…, \(\overrightarrow {{x_n}} \) và các hệ số của chúng.
2. Ví dụ minh hoạ
Cho tứ diện ABCD. Đặt \(\overrightarrow {AB} = \overrightarrow a \), \(\overrightarrow {AC} = \overrightarrow b \), \(\overrightarrow {AD} = \overrightarrow c \). Gọi M là trung điểm của đoạn thẳng BC. Phân tích \(\overrightarrow {DM} \) theo các vecto \(\overrightarrow a \), \(\overrightarrow b \), \(\overrightarrow c \).
Giải:
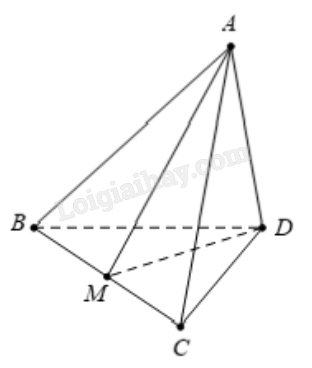
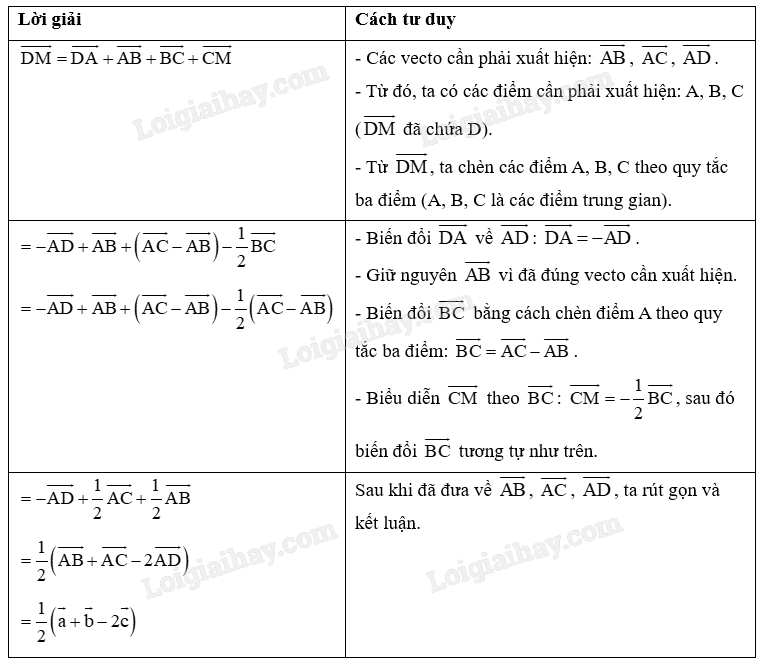
Các bài khác cùng chuyên mục
- Cách tính cường độ hợp lực trong không gian - Toán 12
- Cách chứng minh ba điểm thẳng hàng dựa vào vecto cùng phương trong không gian - Toán 12
- Hệ trục toạ độ. Cách tìm toạ độ điểm và vecto trong không gian - Toán 12
- Cách tìm toạ độ hình chiếu trên trục và mặt phẳng toạ độ - Toán 12
- Cách tìm toạ độ điểm đối xứng qua trục và mặt phẳng toạ độ - Toán 12







